Ted Gibbons
Waterloo, ON, N2J 1Z7
15192414403
Generating the greater reality of one-sidedness
Your Custom Text Here

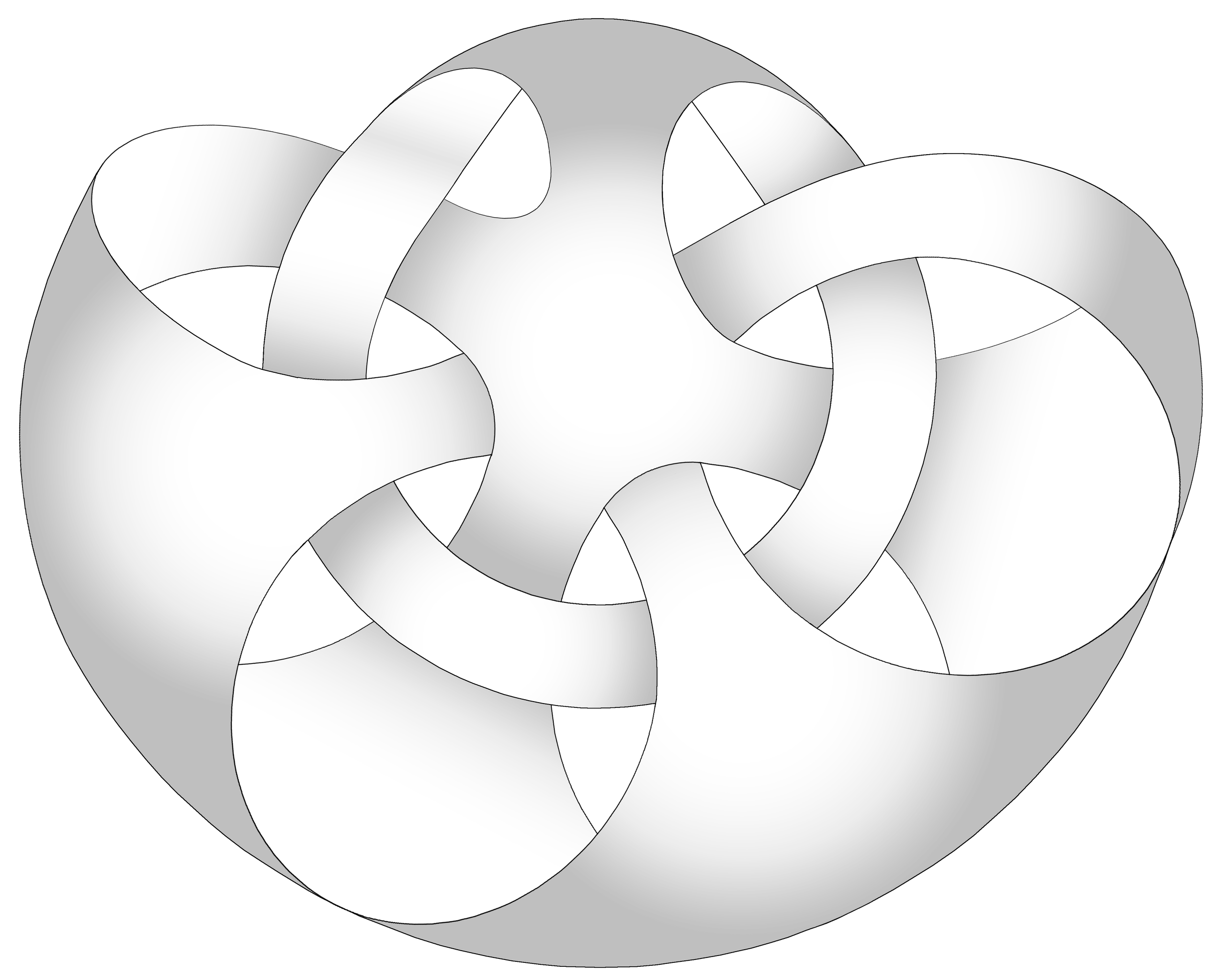
T5 m2 = O b6
The Metamobius algorithm also generates fundamental two-sided surfaces. The T5 m2 = O b6 is a beautiful example of one of these. The algorithm has many variables that can be adjusted to produce an endless variety of one-sided and two-sided surfaces. One option is to produce either a symmetrical or asymmetrical surface. After creating the Metamobius algorithm I spent the first while mainly applying it to symmetrical surfaces. Later I began generating asymmetrical one-sidedness, and I was immediately fascinated with the beauty of broken symmetry within this kind of geometry.

